The speed of light, usually denoted by c, is a physical constant, so named because it is the speed at which light and all other electromagnetic radiation travels in vacuum. Its value is exactly 299,792,458 metres per second,[1][2] often approximated as 300,000 kilometres per second or 186,000 miles per second. In the theory of relativity, c connects space and time in spacetime, and appears in the famous equation of mass–energy equivalence E = mc2.[3] The speed of light is the speed of all massless particles and associated fields in vacuum, and it is believed to be the speed of gravity and of gravitational waves and an upper bound on the speed at which energy, matter, and information can travel.
The speed at which light propagates through transparent materials, such as glass or air, is less than c. The ratio between c and the speed v at which light travels in a material is called the refractive index n of the material (n = c / v). For example, for visible light the refractive index of glass is typically around 1.5, meaning that light in glass travels at c / 1.5 ≈ 200,000 km/s; the refractive index of air for visible light is about 1.0003, so the speed of light in air is very close to c.

Sunlight takes about 8 minutes, 19 seconds to reach Earth.
Exact values | metres per second | 299,792,458 |
| Planck units | 1 |
Approximate values | kilometres per second | 300,000 |
| kilometres per hour | 1,079 million |
| miles per second | 186,000 |
| miles per hour | 671 million |
| astronomical units per day | 173 |
Approximate light signal travel times | Distance | Time |
| one foot | 1.0 ns |
| one metre | 3.3 ns |
| one kilometre | 3.3 μs |
| one statute mile | 5.4 μs |
| from the geostationary orbit to Earth | 119 ms |
| the length of Earth's equator | 134 ms |
| from Moon to Earth | 1.3 s |
| from Sun to Earth (1 AU) | 8.3 min |
| one parsec | 3.26 years |
| from Alpha Centauri to Earth | 4.4 years |
| across the Milky Way | 100,000 years |
| from the Andromeda Galaxy to Earth | 2.5 million years |
For much of human history, it was debated whether light was transmitted instantaneously or
merely very quickly. Ole Rømer first demonstrated that it travelled at a finite speed by studying the apparent motion of Jupiter's moon Io. After centuries of increasingly precise measurements, in 1975 the speed of light was known to be 299,792,458 m/s with a relative measurement uncertainty of 4 parts per billion. In 1983, the metre was redefined in the International System of Units (SI) as the distance travelled by light in vacuum in 1⁄299,792,458 of a second. As a result, the numerical value of c in metres per second is now fixed exactly by the definition of the metre.
Numerical value, notation and units
The speed of light is a dimensional physical constant, so its numerical value depends on the system of units used. In the International System of Units (SI), the metre is defined as the distance light travels in vacuum in 1⁄299,792,458 of a second (see "Increased accuracy and redefinition of the metre", below). The effect of this definition is to fix the speed of light in vacuum at exactly 299,792,458 m/s.[Note 1][5][6][7]
The speed of light in vacuum is usually denoted by c, for "constant" or the Latin celeritas (meaning "swiftness"). Originally, the symbol V was used, introduced by Maxwell in 1865. In 1856, Weber and Kohlrausch had used c for a constant later shown to equal √2 times the speed of light in vacuum. In 1894, Drude redefined c with its modern meaning. Einstein used V in his original German-language papers on special relativity in 1905, but in 1907 he switched to c, which by then had become the standard symbol.[8][9]
Some authors use c for the speed of waves in any material medium, and c0 for the speed of light in vacuum.[10] This subscripted notation, which is endorsed in official SI literature,[1] has the same form as other related constants: namely, μ0 for the vacuum permeability or magnetic constant, ε0 for the vacuum permittivity or electric constant, and Z0 for the impedance of free space. This article uses c exclusively for the speed of light in vacuum.
In branches of physics in which the speed of light plays an important part, such as in relativity, it is common to use systems of natural units of measurement in which c = 1.[11][12] When such a system of measurement is used, the speed of light drops out of the equations of physics, because multiplication or division by 1 does not affect the result.
Fundamental role in physics
See also: Introduction to special relativity and Special relativity
The speed at which light propagates in vacuum is independent both of the motion of the light source and of the inertial frame of reference of the observer.[Note 2] This invariance of the speed of light was postulated by Albert Einstein in 1905, motivated by Maxwell's theory of electromagnetism and the lack of evidence for the luminiferous ether;[13] it has since been consistently confirmed by many experiments.[Note 3][12][14] The theory of special relativity explores the consequences of the existence of such an invariant speed c and the assumption that the laws of physics are the same in all inertial frames of reference.[15][16] One consequence is that c is the speed at which all massless particles and waves, including light, must travel.
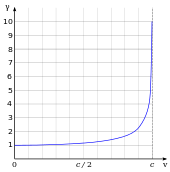
The Lorentz factor
γ as a function of velocity. It starts at 1 and approaches infinity as
v approaches
c.
Special relativity has many counter-intuitive implications, which have been verified in many experiments.[17] These include the equivalence of mass and energy (E = mc2), length contraction (moving objects shorten),[Note 4] and time dilation (moving clocks run slower). The factor γ by which lengths contract and times dilate, known as the Lorentz factor, is given by γ = (1 − v2/c2)−1/2, where v is the speed of the object; its difference from 1 is negligible for speeds much slower than c, such as most everyday speeds—in which case special relativity is closely approximated by Galilean relativity—but it increases at relativistic speeds and diverges to infinity as v approaches c.
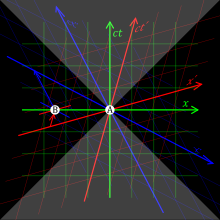
Event A precedes B in the red frame, is simultaneous with B in the green frame, and follows B in the blue frame.
Another counter-intuitive consequence of special relativity is the relativity of simultaneity: if the spatial distance between two events A and B is greater than the time interval between them multiplied by c, then there are frames of reference in which A precedes B, others in which B precedes A, and others in which they are simultaneous; neither event can be the cause of the other.
The results of special relativity can be summarized by treating space and time as a unified structure known as spacetime (with c relating the units of space and time), and requiring that physical theories satisfy a special symmetry called Lorentz invariance, whose mathematical formulation contains the parameter c.[20] Lorentz invariance has become an almost universal assumption for modern physical theories, such as quantum electrodynamics, quantum chromodynamics, the Standard Model of particle physics, and general relativity. As such, the parameter c is ubiquitous in modern physics, appearing in many contexts that may seem to be unrelated to light. For example, general relativity predicts that c is also the speed of gravity and of gravitational waves.[21]
In non-inertial frames of reference (gravitationally curved space or accelerated reference frames), the local speed of light is constant and equal to c, but the speed of light along a trajectory of finite length can differ from c, depending on how distances and times are defined.[22]
It is generally assumed in physics that fundamental constants such as c have the same value throughout spacetime, meaning that they do not depend on location and do not vary with time. However, various theories have suggested that the speed of light has changed over time.[23][24] Although no conclusive evidence for such theories has been found, they remain the subject of ongoing research.[25][26][27]
Upper limit on speeds
According to special relativity, the energy of an object with rest mass m and speed v is given by γmc2, where γ is the Lorentz factor defined above. When v is zero, γ is equal to one, giving rise to the famous E = mc2 formula for mass-energy equivalence. Since the γ factor approaches infinity as v approaches c, it would take an infinite amount of energy to accelerate an object with mass to the speed of light. The speed of light is the upper limit for the speeds of objects with positive rest mass.[28]
More generally, it is normally impossible for any information or energy to travel faster than c. One reason is that according to the theory of special relativity, if something were travelling faster than c relative to an inertial frame of reference, it would be travelling backwards in time relative to another frame,[Note 5] and causality would be violated.[Note 6][30] In such a frame of reference, an "effect" could be observed before its "cause". Such a violation of causality has never been recorded,[14] and would lead to paradoxes.[Note 7][31] Faster-than-light observations and experiments
Main article: Faster-than-light
There are situations in which it may seem that matter, energy, or information travels at speeds greater than c, but they do not. For example, if a laser beam is swept quickly across a distant object, the spot of light can move faster than c, but the only physical entities that are moving are the laser and its emitted light, which travels at the speed c from the laser to the various positions of the spot. The movement of the spot will be delayed after the laser is moved because of the time it takes light to get to the distant object from the laser. Similarly, a shadow projected onto a distant object can be made to move faster than c.[32] In neither case does any matter or information travel faster than light.[33]
In some interpretations of quantum mechanics, certain quantum effects may seem to be transmitted faster than c—and thus instantaneously in some frame—as in the EPR paradox. An example involves the quantum states of two particles that can be entangled. Until either of the particles is observed, they exist in a superposition of two quantum states. If the particles are separated and one particle's quantum state is observed, the other particle's quantum state is determined instantaneously (i.e., faster than light could travel from one particle to the other). However, it is impossible to control which quantum state the first particle will take on when it is observed, so information cannot be transmitted in this manner.[33][34]
Another quantum effect that predicts the occurrence of faster-than-light speeds is called the Hartman effect; under certain conditions the time needed for a particle to tunnel through a barrier is constant.[35][36] This could result in a particle crossing a large gap faster-than-light. However, no information can be sent using this effect.[37]
As is discussed in the propagation of light in a medium section below, many wave velocities can exceed c. For example, the phase velocity of X-rays through most glasses can routinely exceed c,[38] but such waves cannot convey any information.[39]
The rate of change in the distance between two objects in a frame of reference with respect to which both are moving (their closing speed) may have a value in excess of c. However, this does not represent the speed of any single object as measured in a single inertial frame.[citation needed]
So-called superluminal motion is seen in certain astronomical objects,[40] such as the relativistic jets of radio galaxies and quasars. However, these jets are not moving at speeds in excess of the speed of light: the apparent superluminal motion is a projection effect caused by objects moving near the speed of light and approaching Earth at a small angle to the line of sight: since the light which was emitted when the jet was farther away took longer to reach the Earth, the time between two successive observations corresponds to a longer time between the instants at which the light rays were emitted.[41]
In models of the expanding universe, the farther galaxies are from each other, the faster they drift apart. This receding is not due to motion through space, but rather to the expansion of space itself.[33] For example, galaxies far away from Earth appear to be moving away from the Earth with a speed proportional to their distances. Beyond a boundary called the Hubble sphere, this apparent recessional velocity becomes greater than the speed of light.[42]
Propagation of light
In classical physics, light is described as a type of electromagnetic wave. The classical behaviour of the electromagnetic field is described by Maxwell's equations, which predict that the speed c with which electromagnetic waves (such as light) propagate through the vacuum is related to the electric constant ε0 and the magnetic constant μ0 by the equation c = 1/√ε0μ0.[43]
In modern quantum physics, the electromagnetic field is described by the theory of quantum electrodynamics (QED). In this theory, light is described by the fundamental excitations (or quanta) of the electromagnetic field, called photons. In QED, photons are massless particles and thus, according to special relativity, they must travel at the speed of light.
Extensions of QED in which the photon has a mass have been considered. In such a theory, its speed would depend on its frequency, and the invariant speed c of special relativity would then be the upper limit of the speed of light in vacuum.[22] To date no such effects have been observed,[44][45][46] putting stringent limits on the mass of the photon. The limit obtained depends on the used model: if the massive photon is described by Proca theory,[47] the experimental upper bound for its mass is about 10−57 grams;[48] if photon mass is generated by a Higgs mechanism, the experimental upper limit is less sharp, m ≤ 10−14 eV/c2 [47] (roughly 2 × 10−47 g).
Another reason for the speed of light to vary with its frequency would be the failure of special relativity to apply to arbitrarily small scales, as predicted by some proposed theories of quantum gravity. In 2009, the observation of the spectrum of gamma-ray burst GRB 090510 did not find any difference in the speeds of photons of different energies, confirming that Lorentz invariance is verified at least down to the scale of the Planck length (lP = √ħG/c3 ≈ 1.6163×10−35 m) divided by 1.2.[49]
In a medium
See also: Refractive index and Dispersion (optics)
When light enters materials, its energy is absorbed. In the case of transparent materials, this energy is quickly re-radiated. However, this absorption and re-radiation introduces a delay. As light propagates through dielectric material it undergoes continuous absorption and re-radiation. Therefore the speed of light in a medium is said to be less than c, which should be read as the speed of energy propagation at the macroscopic level. At an atomic level, electromagnetic waves always travel at c in the empty space between atoms. Two factors influence this slowing: stronger absorption leading to shorter path length between each re-radiation cycle, and longer delays. The slowing is therefore the result of these two factors.[50] The refractive index of a transparent material is defined as the ratio of c to the speed of light v in the material. Larger indices of refraction indicate smaller speeds. The refractive index of a material may depend on the light's frequency, intensity, polarization, or direction of propagation. In many cases, though, it can be treated as a material-dependent constant. The refractive index of air is approximately 1.0003.[51] Denser media, such as water and glass, have refractive indexes of around 1.3 and 1.5 respectively for visible light.[52]Diamond has a refractive index of about 2.4.[53]

The light passing through a dispersive prism demonstrates refraction and, by the splitting of white light into a spectrum of colors, dispersion.
If the refractive index of a material depends on the frequency of the light passing through the medium, there exist two notions of the speed of light in the medium. One is the speed of a wave of a single frequency f. This is called the phase velocity vp(f), and is related to the frequency-dependent refractive index n(f) by vp(f) = c/n(f). The other is the average velocity of a pulse of light consisting of different frequencies of light. This is called the group velocity and not only depends on the properties of the medium but also the distribution of frequencies in the pulse. A pulse with different group and phase velocities is said to undergo dispersion.
Certain materials have an exceptionally low group velocity for light waves, a phenomenon called slow light. In 1999, a team of scientists led by Lene Hau were able to slow the speed of a light pulse to about 17 metres per second (61 km/h; 38 mph);[54] in 2001, they were able to momentarily stop a beam.[55] In 2003, scientists at Harvard University and the Lebedev Physical Institute in Moscow, succeeded in completely halting light by directing it into a Bose–Einstein condensate of the element rubidium, the atoms of which, in Lukin's words, behaved "like tiny mirrors" due to an interference pattern in two "control" beams.[56][57]
It is also possible for the group velocity of light pulses to exceed c.[58] In an experiment in 2000, laser beams travelled for extremely short distances through caesium atoms with a group velocity of 300 times c.[59] It is not possible to transmit information faster than c by this means because the speed of information transfer cannot exceed the front velocity of the wave pulse, which is always less than c.[60] The requirement that causality is not violated implies that the real and imaginary parts of the dielectric constant of any material, corresponding respectively to the index of refraction and to the attenuation coefficient, are linked by the Kramers–Kronig relations.[61]
Practical effects of finiteness
The finiteness of the speed of light has implications for various sciences and technologies. For some it creates challenges or limits: for example, c, being the upper limit of the speed with which signals can be sent, provides a theoretical upper limit for the operating speed of microprocessors. For others it creates opportunities, for example to measure distances.
The speed of light is of relevance to communications. For example, given the equatorial circumference of the Earth is about 40,075 km and c about 300,000 km/s, the theoretical shortest time for a piece of information to travel half the globe along the surface is about 67 milliseconds. When light is traveling around the globe in an optical fiber, the actual transit time is longer, in part because the speed of light is slower by about 35% in an optical fiber, depending on its refractive index n.[62] Furthermore, straight lines rarely occur in global communications situations, and delays are created when the signal passes through an electronic switch or signal regenerator.[63]

A beam of light is depicted travelling between the Earth and the Moon in the same time it takes light to scale the distance between them: 1.255 seconds at its mean orbital (surface to surface) distance. The relative sizes and separation of the Earth–Moon system are shown to scale.
Another consequence of the finite speed of light is that communications between the Earth and spacecraft are not instantaneous. There is a brief delay from the source to the receiver, which becomes more noticeable as distances increase. This delay was significant for communications between ground control and Apollo 8 when it became the first manned spacecraft to orbit the Moon: for every question, the ground control station had to wait at least three seconds for the answer to arrive.[64] The communications delay between Earth and Mars is almost ten minutes. As a consequence of this, if a robot on the surface of Mars were to encounter a problem, its human controllers would not be aware of it until ten minutes later; it would then take at least a further ten minutes for instructions to travel from Earth to Mars.
The speed of light can also be of concern over very short distances. In supercomputers, the speed of light imposes a limit on how quickly data can be sent between processors. If a processor operates at 1 gigahertz, a signal can only travel a maximum of about 30 centimetres (1 ft) in a single cycle. Processors must therefore be placed close to each other to minimize communication latencies, which can cause difficulty with cooling. If clock frequencies continue to increase, the speed of light will eventually become a limiting factor for the internal design of single chips.[65]
Distance measurement
Radar systems measure the distance to a target by the time it takes a radio-wave pulse to return to the radar antenna after being reflected by the target: the distance to the target is half the round-trip transit time multiplied by the speed of light. A Global Positioning System (GPS) receiver measures its distance to GPS satellites based on how long it takes for a radio signal to arrive from each satellite, and from these distances calculates the receiver's position. Because light travels about 300,000 kilometres (186,000 miles) in one second, these measurements of small fractions of a second must be very precise. The Lunar Laser Ranging Experiment, radar astronomy and the Deep Space Network determine distances to the Moon, planets and spacecraft, respectively, by measuring round-trip transit times.
Astronomy
The finite speed of light is important in astronomy. Due to the vast distances involved, it can take a very long time for light to travel from its source to Earth. For example, it has taken 13 billion (13 × 109) years for light to travel to Earth from the faraway galaxies viewed in the Hubble Ultra Deep Field images.[66][67] Those photographs, taken today, capture images of the galaxies as they appeared 13 billion years ago, when the universe was less than a billion years old.[66] The fact that farther-away objects appear younger (due to the finite speed of light) allows astronomers to infer the evolution of stars, of galaxies, and of the universe itself.
Astronomical distances are sometimes expressed in light-years, especially in popular science publications.[68] A light‑year is the distance light travels in one year, around 9461 billion kilometres, 5879 billion miles, or 0.3066 parsecs. Proxima Centauri, the closest star to Earth after the Sun, is around 4.2 light‑years away.[69]
Measurement
To measure the speed of light, various methods can be used which involve observation of astronomical phenomena or experimental setups on Earth. The setups could use mechanical devices (e.g. toothed wheels), optics (e.g. beam splitters, lenses and mirrors), electro-optics (e.g. lasers), or electronics in conjunction with a cavity resonator.
Astronomical measurements
Due to the large scale and the vacuum of space observations in the solar system and in astronomy in general provide a natural setting for measuring the speed of light. The result of such a measurement usually appears as the time needed for light to transverse some reference distance in the solar system such as the radius of the Earth's orbit. Historically such measurements could be made fairly accurately, compared to how accurate the length of the reference distance is known in Earth-based units. As such, it is customary to express the results in astronomical units per day. An astronomical unit is approximately equal to the average distance between the Earth and the Sun.[Note 8] Since the most used reference length scale in modern experiments (the SI metre) is determined by the speed of light, the value of c is fixed when measured in metres per second. Measurements of c in astronomical units provides an independent alternative to measure c.
One such method was used by Ole Christensen Rømer to provide the first quantitative estimate of the speed of light.[71][72] When observing the periods of moons orbiting a distant planet these periods appear to be shorter when the Earth is approaching that planet than when the Earth is receding from it. This effect occurs because the Earth's movement causes the path travelled by light from the planet to Earth to shorten (or lengthen respectively). The observed change in period is the time needed by light to cover the difference in path length. Rømer observed this effect for Jupiter's innermost moon Io and deduced from it that light takes 22 minutes to cross the diameter of the Earth's orbit.

Aberration of light: light from a distant source will appear to a different location for a moving telescope due to the finite speed of light.
Another method is to use the aberration of light, discovered and explained by James Bradley in the 18th century.[73] This effect results from the vector addition of the velocity of light arriving from a distant source (such as a star) and the velocity of its observer (see diagram on the left). A moving observer thus sees the light coming from a slightly different direction and consequently sees the source at a position shifted from its original position. Since the direction of the Earth's velocity changes continuously as the Earth orbits the Sun, this effect causes the apparent position of stars to move around. From the angular difference in the position of stars (maximally 20.5 arcseconds)[74] it is possible to express the speed of light in terms of the Earth's velocity around the Sun, which with the known length of a year can be easily converted in the time needed to travel from the Sun to Earth. In 1729, Bradley used this method to derive that light travelled 10,210 times faster than the Earth in its orbit (the modern figure is 10,066 times faster) or, equivalently, that it would take light 8 minutes 12 seconds to travel from the Sun to the Earth.[73]
Nowadays, the "light time for unit distance"—the inverse of c, expressed in seconds per astronomical unit—is measured by comparing the time for radio signals to reach different spacecraft in the Solar System, with their position calculated from the gravitational effects of the Sun and various planets. By combining many such measurements, a best fit value for the light time per unit distance is obtained. As of 2009[update], the best estimate, as approved by the International Astronomical Union (IAU), is:[75][76][77]
- light time for unit distance: 499.004783836(10) s
- c = 0.00200398880410(4) AU/s = 173.144632674(3) AU/day
The relative uncertainty in these measurements is 0.02 parts per billion (2 × 10−11), equivalent to the uncertainty in Earth-based measurements of length by interferometry.[78][79] Since the meter is defined to be the length travelled by light in a certain time interval, the measurement of the light time for unit distance can also be interpreted as measuring the length of an AU in meters.
Time of flight techniques
A method of measuring the speed of light is to measure the time needed for light to travel to a mirror at a known distance and back. This is the working principle behind the Fizeau–Foucault apparatus developed by Hippolyte Fizeau and Léon Foucault.
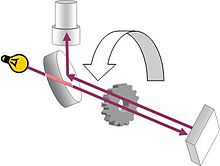
Diagram of the Fizeau apparatus
The setup as used by Fizeau consists of a beam of light directed at a mirror 8 kilometres (5 mi) away. On the way from the source to the mirror, the beam passes through a rotating cogwheel. At a certain rate of rotation, the beam passes through one gap on the way out and another on the way back, but at slightly higher or lower rates, the beam strikes a tooth and does not pass through the wheel. Knowing the distance between the wheel and the mirror, the number of teeth on the wheel, and the rate of rotation, the speed of light can be calculated.[80]
The method of Foucault replaces the cogwheel by a rotating mirror. Because the mirror keeps rotating while the light travels to the distant mirror and back, the light is reflected from the rotating mirror at a different angle on its way out than it is on its way back. From this difference in angle, the known speed of rotation and the distance to the distant mirror the speed of light may be calculated.[81]
Nowadays, using oscilloscopes with time resolutions of less than one nanosecond, the speed of light can be directly measured by timing the delay of a light pulse from a laser or an LED reflected from a mirror. This method is less precise (with errors of the order of 1%) than other modern techniques, but it is sometimes used as a laboratory experiment in college physics classes.[82][83][84]
Electromagnetic constants
An option for measuring c that does not directly depend on the propagation of electromagnetic waves is to use relation between c and the vacuum permittivity ε0 vacuum permeability μ0 established by Maxwell theory: c2 = 1/ε0μ0. The vacuum permittivity may be determined by measuring the capacitance and dimensions of a capacitor, whereas the value of the vacuum permeability is fixed at exactly 4π×10−7 H·m−1 through the definition of the ampere. Rosa and Dorsey used this method in 1907 to find a value of 299,710±22 km/s.[85][86]
Cavity resonance

Electromagnetic
standing waves in a cavity.
Another way to measure the speed of light is to independently measure the frequency f and wavelength λ of an electromagnetic wave in vacuum. The value of c can then be found by using the relation c = fλ. One option is to measure the resonance frequency of a cavity resonator. If the dimensions of the resonance cavity are also known, these can be used determine the wavelength of the wave. In 1946, Louis Essen and A.C. Gordon-Smith establish the frequency for a variety of normal modes of microwaves of a microwave cavity of precisely known dimensions. As the wavelength of the modes was known from the geometry of the cavity and from electromagnetic theory, knowledge of the associated frequencies enabled a calculation of the speed of light.[85][87]
The Essen–Gordon-Smith result, 299,792±9 km/s, was substantially more precise than those found by optical techniques.[85] By 1950, repeated measurements by Essen established a result of 299,792.5±3.0 km/s.[88]
A household demonstration of this technique is possible, using a microwave oven and food such as marshmallows or margarine: if the turntable is removed so that the food does not move, it will cook the fastest at the antinodes (the points at which the wave amplitude is the greatest), where it will begin to melt. The distance between two such spots is half the wavelength of the microwaves; by measuring this distance and multiplying the wavelength by the microwave frequency (usually displayed on the back of the oven, typically 2450 MHz), the value of c can be calculated, "often with less than 5% error".[89][90]
Laser interferometry
An alternative to the cavity resonator method to find the wavelength for determining the speed of light is to use a form of interferometer.[91] A coherent light beam with a known frequency (f), as from a laser, is split to follow two paths and then recombined. By carefully changing the path length and observing the interference pattern, the wavelength of the light (λ) can be determined, which is related to the speed of light by the equation c = λf.
The main difficulty in measuring c through interferometry is to measure the frequency of light in or near the optical region; such frequencies are too high to be measured with conventional methods. This was first overcome by a group at the US National Institute of Standards and Technology (NIST) laboratories in Boulder, Colorado, in 1972.[92] By a series of photodiodes and specially constructed metal–insulator–metal diodes, they succeeded in linking the frequency of a methane-stabilized infrared laser to the frequency of the caesium transition used in atomic clocks (nearly 10,000 times lower, in the microwave region).[93] Their results for the frequency and wavelength of the infrared laser, and the resulting value for c, were:
- f = 88.376181627±0.000000050 THz;
- λ = 3.392231376±0.000000012 µm;
- c = 299,792,456.2±1.1 m/s;
nearly a hundred times more precise than previous measurements of the speed of light.[92][93]
History
Until the early modern period, it was not known whether light travelled instantaneously or at a finite speed. The first extant recorded examination of this subject was in ancient Greece. Empedocles was the first to claim that the light has a finite speed.[94] He maintained that light was something in motion, and therefore must take some time to travel. Aristotle argued, to the contrary, that "light is due to the presence of something, but it is not a movement".[95] Euclid and Ptolemy advanced the emission theory of vision, where light is emitted from the eye, thus enabling sight. Based on that theory, Heron of Alexandria argued that the speed of light must be infinite because distant objects such as stars appear immediately upon opening the eyes.
Early Islamic philosophers initially agreed with the Aristotelian view that light had no speed of travel. In 1021, Islamic physicist Alhazen (Ibn al-Haytham) published the Book of Optics, in which he used experiments related to the camera obscura to support the now accepted intromission theory of vision, in which light moves from an object into the eye.[96] This led Alhazen to propose that light must therefore have a finite speed,[95][97][98] and that the speed of light is variable, decreasing in denser bodies.[98][99] He argued that light is a "substantial matter", the propagation of which requires time "even if this is hidden to our senses".[100]
Also in the 11th century, Abū Rayhān al-Bīrūnī agreed that light has a finite speed, and observed that the speed of light is much faster than the speed of sound.[101] Roger Bacon argued that the speed of light in air was not infinite, using philosophical arguments backed by the writing of Alhazen and Aristotle.[102][103] In the 1270s, Witelo considered the possibility of light travelling at infinite speed in a vacuum, but slowing down in denser bodies.[104]
In the early 17th century, Johannes Kepler believed that the speed of light was infinite, since empty space presents no obstacle to it. René Descartes argued that if the speed of light were finite, the Sun, Earth, and Moon would be noticeably out of alignment during a lunar eclipse. Since such misalignment had not been observed, Descartes concluded the speed of light was infinite. Descartes speculated that if the speed of light were found to be finite, his whole system of philosophy might be demolished.[95]
First measurement attempts
In 1629, Isaac Beeckman proposed an experiment in which a person would observe the flash of a cannon reflecting off a mirror about one mile (1.6 km) away. In 1638, Galileo Galilei proposed an experiment, with an apparent claim to having performed it some years earlier, to measure the speed of light by observing the delay between uncovering a lantern and its perception some distance away. He was unable to distinguish whether light travel was instantaneous or not, but concluded that if it weren't, it must nevertheless be extraordinarily rapid.[105][106] Galileo's experiment was carried out by the Accademia del Cimento of Florence, Italy, in 1667, with the lanterns separated by about one mile, but no delay was observed. Based on the modern value of the speed of light, the actual delay in this experiment would be about 11 microseconds. Robert Hooke explained the negative results as Galileo had by pointing out that such observations did not establish the infinite speed of light, but only that the speed must be very great.

Rømer's observations of the occultations of Io from Earth
The first quantitative estimate of the speed of light was made in 1676 by Ole Christensen Rømer (see Rømer's determination of the speed of light).[71][72] From the observation that the periods of Jupiter's innermost moon Io appeared to be shorter when the earth was approaching Jupiter than when receding from it, he concluded that light travels at a finite speed, and was able to estimate that would take light 22 minutes to cross the diameter of Earth's orbit. Christiaan Huygens combined this estimate with an estimate for the diameter of the Earth's orbit to obtain an estimate of speed of light of 220,000 km/s, 26% lower than the actual value.[107]
In his 1704 book Opticks, Isaac Newton reported Rømer's calculations of the finite speed of light and gave a value of "seven or eight minutes" for the time taken for light to travel from the Sun to the Earth (the modern value is 8 minutes 19 seconds).[108] Newton queried whether Rømer's eclipse shadows were coloured; hearing that they weren't, he concluded the different colours travelled at the same speed. In 1729, James Bradley discovered the aberration of light.[73] From this effect he determined that light must travel 10,210 times faster than the Earth in its orbit (the modern figure is 10,066 times faster) or, equivalently, that it would take light 8 minutes 12 seconds to travel from the Sun to the Earth.[73]
19th and early 20th century
In the 19th century Hippolyte Fizeau developed a method to determine the speed of light based on time-of-flight measurements on Earth and reported a value of 315,000 km/s. His method was improved upon by Léon Foucault who obtained a value of 298,000 km/s in 1862.[80] James Clerk Maxwell observed that this value was very close to the parameter c appearing in his theory of electromagnetism as the propagation speed of the electromagnetic field, and suggested that light was an electromagnetic wave.[109]
It was thought at the time that electromagnetic field existed in some background medium called the luminiferous aether. This aether acted as an absolute reference frame for all physics and should be possible to measure the motion of the Earth with respect to this medium. In the second half of the 19th century and the beginning of the 20th century several experiments were performed to try to detect this motion, the most famous of which is the experiment performed by Albert Michelson and Edward Morley in 1887.[110] None of the experiments found any hint of the motion, finding that the speed of light was the same in every direction.[111]
In 1905 Albert Einstein proposed that the speed of light was independent of the motion of the source or observer. Using this and the principle of relativity as a basis he derived his special theory of relativity, in which the speed of light c featured as a fundamental parameter, also appearing in contexts unrelated to light.[112]
Increased accuracy and redefinition of the metre
See also: Metre
History of measurements of c | Year | Author and method | Value (km/s) |
| 1675 | Rømer and Huygens, moons of Jupiter | 220,000[72][107] |
| 1729 | James Bradley, aberration of light | 301,000 |
| 1849 | Hippolyte Fizeau, toothed wheel | 315,000 |
| 1862 | Léon Foucault, rotating mirror | 298,000±500 |
| 1907 | Rosa and Dorsay, EM constants | 299,788±30 |
| 1926 | Albert Michelson, rotating mirror | 299,796±4 |
| 1947 | Essen and Gordon-Smith, cavity resonator | 299,792±3 |
| 1958 | K.D. Froome, radio interferometry | 299,792.5±0.1 |
| 1973 | Evanson et al., laser interferometry | 299,792.4574±0.001 |
| 1983 | 17th CGPM, definition of the metre | 299,792.458 (exact) |
In the second half of the 20th century much progress was made in increasing the accuracy of measurements of the speed of light, first by cavity resonance techniques and later by laser interferometer techniques. In 1972, using the latter method, a team at the US National Institute of Standards and Technology (NIST) laboratories in Boulder, Colorado determined the speed of light to be c = 299,792,456.2±1.1 m/s.[92][93] Almost all the uncertainty in this measurement of the speed of light was due to uncertainty in the length of the metre.[93][92][113] Since 1960, the metre had been defined as a given number of wavelengths of the light of one of the spectral lines of krypton,[Note 9] but it turned out that the chosen spectral line was not perfectly symmetrical.[93] This made its wavelength, and hence the length of the metre, uncertain, because the definition did not specify what point on the line profile (e.g., its maximum-intensity point or its centre of gravity) it referred to.[Note 10]
To get around this problem, in 1975, the 15th Conférence Générale des Poids et Mesures (CGPM) recommended using 299,792,458 metres per second for "the speed of propagation of electromagnetic waves in vacuum".[113] Based on this recommendation, the 17th CGPM in 1983 redefined the metre as "the length of the path travelled by light in vacuum during a time interval of 1⁄299,792,458 of a second".[115]
The effect of this definition gives the speed of light the exact value 299,792,458 m/s, which is nearly the same as the value 299,792,456.2±1.1 m/s obtained in the 1972 experiment. The CGPM chose this value to minimise any change in the length of the metre.[116][117] As a result, in the SI system of units the speed of light is now a defined constant.[7] Improved experimental techniques do not affect the value of the speed of light in SI units, but do result in a more precise realisation of the SI metre.[118][119]
 is the fluid density,
is the fluid density, is the substantive derivative (also called the material derivative),
is the substantive derivative (also called the material derivative),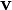 is the velocity vector,
is the velocity vector, is the body force vector, and
is the body force vector, and is a tensor that represents the surface forces applied on a fluid particle (the comoving stress tensor).
is a tensor that represents the surface forces applied on a fluid particle (the comoving stress tensor). is a symmetric tensor. In general, (in three dimensions)
is a symmetric tensor. In general, (in three dimensions)  has the form:
has the form: are normal stresses,
are normal stresses, are tangential stresses (shear stresses).
are tangential stresses (shear stresses). is the velocity gradient perpendicular to the direction of shear
is the velocity gradient perpendicular to the direction of shear
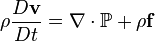





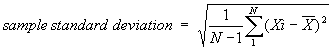





 and the wavelength by λ =
and the wavelength by λ =  , where c is the speed of light in vacuum.
, where c is the speed of light in vacuum.
 is the reduced Planck's constant (Planck's constant divided by 2
is the reduced Planck's constant (Planck's constant divided by 2








